18.02 Practice Exam 3 -- April, 1999

Directions: Suggested time: 70 minutes.

Problem 1 (15 points) The solid $R$ is the piece of the first octant cut off by the plane

$$x+y+z=1$$

Set up an iterated double integral in rectangular coordinates which gives the volume of $R$. (Give the integrand and limits of integration, but do not evaluate.)

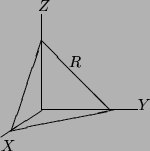
Problem 2 (15 points) A circular disc has radius $a$ and $A$ is a point on its circumference. The density at any point $P$ on the disc is equal to the distance of $P$ from $A$. Set up an iterated double integral in polar coordinates which gives the mass of the disc. Place $A$ at the origin. (Give the integrand and limits, but to not evaluate the integral.)

Problem 3 (15 points) Evaluate the integral $\int^1_0 \int^1_x \cos (y^2) \, dy \, dx$ by changing the order of integration. (Sketch the region of integration first.)

Problem 4 (10 points) Change $\int^1_0 \int^x_{-x} (x^2 + y^2)^{3/2} \, dy \, dx$ to an interated integral in polar coordinates. (Do not evaluate it.)

Problem 5 (30 points; 10 each)

a)

${\bf F}= (axy+y^2){\bf i}+ (x^2+bxy+1){\bf j}$; $a,b$ are constants. Show that $F$ is conservative $\Longleftrightarrow a=2$, $b=2$.

b)

Taking $a=2$, $b=2$, find $f(x,y)$ so that ${\bf F}= {\bf\nabla} f$.

c)

Still taking $a=2$, $b=2$, show $\int_C {\bf F} \cdot {\bf dr}=0$ for any curve $C$ beginning and ending on the $x$-axis.

Problem 6 (30 points; 15, 5, 10)

a)

Evaluate $\oint_C -x^2 y \, dx + xy^2 \, dy$ by Green's theorem, if $C$ is the closed curve as pictured passing through $(1,0),$ $(\frac1{\sqrt2},\frac1{\sqrt2}),$ $(0,0),$ and back to $(1,0).$

b)

Show that for any simple closed curve $C$ directed positively,

$$\oint_C -y \, dx = \hbox{Area inside } C \, .$$

c)

The curve $y^2=x^2(1-x)$ shown is given parametrically by

$$x=1-t^2 \, , \quad y=t-t^3 \, .$$

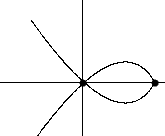
Find the area inside the loop.

Problem 7 (15 points; 5, 10)

a)

Write down in rectangular coordinates the field ${\bf F}=M{\bf i}+N{\bf j}$ whose vectors all have unit length and point radially outward from the origin. (${\bf F}=0$ at $(0,0)$.)

b)

For this field, give the values of $\int_C {\bf F} \cdot{\bf dr}$ over the following curves (no calculation is required):

$C_1$ is the unit semi-circle in the upper half-plane, running from $(1,0)$ to $(-1,0)$

$C_2$ is the line segment from $(0,0)$ to $(1,1)$

Brief solutions.

Problem 1

Thus the volume is $\int^1_0 \int^{1-x}_0 (1-x-y) \, dx \, dy$ OR $\int^1_0 \int^{1-4}_0 (1-x-y) \, dx \, dy.$

Problem 2

With the center on the x-axis

$$\hbox{Mass } = \int^{\pi /2}_{- \pi /2} \int^{2 \cos \theta}_0 r \cdot r \, dr \, d \theta$$

OR with the center on the y-axis:

$$\hbox{Mass } = \int^{\pi}_0 \int^{2a \sin \theta}_0 r \cdot r \, dr \, d \theta$$

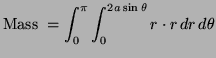
Problem 3 The region of integration for $\int^1_0 \int^1_x$ is

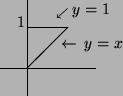
so the integral becomes $\int^1_0 \int^y_0 \cos (y^2) \, dx \, dy$, which evaluates by

 		 Inner: 		 

$\left. \cos (y^2) \cdot x\right]^1_0 = \cos (y^2) \cdot y$

		 Outer: 		 

$\left. \frac{1}{2} \sin (y^2) \right]^1_0 =\frac{1}{2} \sin 1$

Problem 4 The region of integration for $\int^1_0 \int^x_{-x} \ldots \, dy \, dx$ is $(r^2=x^2+y^2)$

so in polar coordinates the integral becomes

$$\int^{\pi / 4}_{- \pi /4}\int^{1\sec \theta)}_0 r^3\times r \, dr \, d \theta.$$

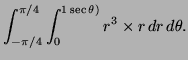
Problem 5

1.

$\vec{F}$ conservative $\Longleftrightarrow \frac{\partial (axy+y^2)}{\partial y} = \frac{\partial (... ...artial x} \Longleftrightarrow ax+2y=2x+by \quad \Longleftrightarrow a=2, \, b=2$

2.

Method 1:

$f(x_1,y_1)= \int_{1}+ \int_2 \qquad \int_1 = 0$ since along $1$ $y=0$ $dy=0$

$\int_2 = \int^y_0 (x^2_1 + 2x_1 y+1) \, dy = x^2_1y_1 + x_1y^2_1 + y_1$

since $x<x_1\, , dx=0$ along path $2$

OR

3.

Method 2: $\frac{\partial f}{\partial x} = 2xy+y^2$

$$% latex2html id marker 986 \begin{array}{rclll} \therefore f... ...g'(y) &=& x^2+2xy+1\\ & &= g'(y) = 1, \quad g(y)=y \end{array}$$

and so

$$f=x^2 y+xy^2 + y$$

4.

Using fundamental theorems:

$$\int^{(x_{0},0)}_{(x_1, 0)} \vec{F} \cdot d \vec{r} = \int^{(x_{0},0)}_{(x_1, 0)}{\bf\nabla}(x^2y+xy^2+y)\cdot d{\vec{r}}= 0-0=0$$

OR

Since $\vec{F}$ is path-independent, we can replace $C$ by a path $D$ on the $x$-axis:

$$\int_C \vec{F} \cdot d\vec{r} = \int_D \vec{F} \cdot d\vec{r} = \int^{x_2}_{x_1} 0 \, (\hbox{since }y=0 \hbox{ on }D) \cdot \, dx = 0.$$

Problem 6

1.

$\oint_C -x^2 y \, dx + xy^2 \, dy = \iint_R y^2-(-x^2) \, dA$ by Green's theorem

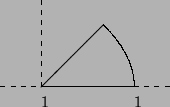
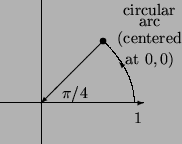
$$= \int^{\pi / 4}_0 \int^1_0 r^2 \cdot r\, dr\,d \theta = \left. \frac{\pi}{4} \cdot \frac{r^4}{4} \right]^1_0 = \frac{\pi}{16}.$$

The original picture can be interpreted to mean that $C$ was not closed, with the part in the x-axis missing. Since this actually contributes nothing to the line integral, the answer is the same!

2.

By Green's theorem, $\oint_C -y \, dx = \iint_R - \frac{\partial(-y)}{\partial y} \, dA = \iint_R \, dA =$ area of $R$

3.

Using (b), area inside loop

$$= \oint -ydx = \int^1_{-1} - (t-t^3)(-2t) \, dt = \int^1_{-1}(2t^2-2t^4) \, dt$$

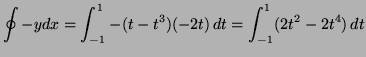$$= \left. \frac{2t^3}{3}- \frac{2t^5}{5} \right]^1_{-1} = \frac{2}{3} - \frac{2}{5} -(\frac{-2}{3} + \frac{2}{5}) = 8/15$$

Problem 7

a)

${\bf F}= \frac{x {\bf i} + y {\bf j}}{\sqrt{x^2+y^2}}$ has magnitude $=1$ (since $\left\vert x {\bf i} + y {\bf j} \right\vert = \sqrt{x^2 + y^2})$ and has radially outward direction.

b)

For $C_1,$ the unit semi-circle in the upper half-plane, running from $(1,0)$ to $(-1,0),$ ${\bf F}$ is perpendicular to the tangent of the curve, at every point, so the integral is zero.

For $C_2,$ ${\bf F}$ and $C$ have the same direction and both are constant (on $C_2)$, so the work is simply $\left\vert{\bf F} \right\vert\times$ (distance) $= 1 \cdot \sqrt{2} = \sqrt{2}.$